Incumbency
October 3, 2020
Background
While there is evidence to suggest that voters don’t select an incumbent just because they are an incumbent, there are various theories that suggest the institutional advantages that an incumbent President has can affect voters’ preferences.
In a randomized study by Adam Brown, it is shown that at the ballot, having “incumbent” next to a candidate’s name does not increase their vote share. In this way, voters do not care about incumbency qua incumbency (i.e. just the state of being an incumbent does not sway voters). However, this study does not investigate certain advantages from which incumbents benefit.
Some of these advantages include, but are not limited to:
- Media Attention: Incumbents have the added benefit of increased media attention throughout their presidency, as compared to challenger’s media attention primarily during the election cycle.
- Fundraising Time: Incumbents can start fundraising the minute they are elected. Challengers on the other hand, do not typically even decide to run for the presidency until about two years before the election.
- No Primary: Incumbents are typically not challenged by a member of their own party. This means that they don’t have to spend money against same-party rivals like challengers do, and they can rally the support of their entire party, whereas challengers must compete against rival factions within their party.
- Economic Manipulation: There is evidence to suggest that incumbents may manipulate the economy to ensure their victory by moving economic gains to election cycles (Achen and Bartels). Incumbents may also allocate funds to competitive states during elections years, in order to possibly influence votes in key states (Kriner and Reeves).
Time For Change Model
The Time For Change Model rewards incumbent parties who have higher approval ratings, higher Q2 GDP Growth, and have only served 1 consecutive term.
Guided by Pollyvote’s recreation of Abramowitz’s Time For Change Model, I used available data from Gallup, The US Bureau of Economic Analysis – Department of Commerce, and former national elections from 1948-2016 in my training set. Abramowitz uses all prior years to an election to train his data, so I decided to do the same.
The Time For Change Model uses three variables to predict the Incumbent Party Vote Share (%):
- Net Approval Rating (%): Measured by approval rating - disapproval rating from Gallup. Abramowitz used approval rating polls from June/July, so I used the most recent poll from either of those months for each election year in my training set.
- Q2 GDP Growth (%): Measured by the rate of change in GDP from Q1 to Q2.
- First Term: Dummy variable to indicate whether or not the incumbent is a first-term president (1) or not (0). Essentially, if a party has been in power for 2 or more consecutive terms, this will be coded as a 0.
Using training data from 1948-2016, the model is summarize below:
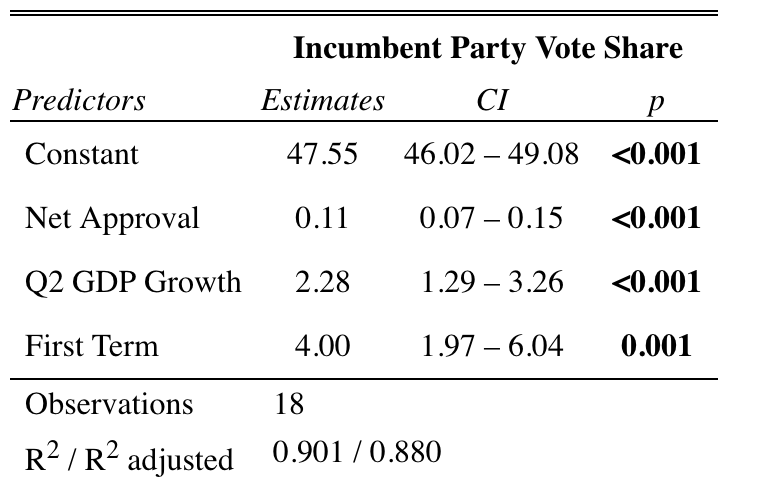
Using the coefficients, this gives us the equation:
(Predicted Incumbent Party Vote Share) = 47.55 + 0.11 * (Net Approval Rating) + 2.28 * (Q2 GDP Growth) + 4 * (First Term)
- For each additional percent in Net Approval Rating, the Incumbent Party Vote Share is predicted to increase by 0.11 percentage points.
- For each additional percent in Q2 GDP Growth, the Incumbent Party Vote Share is predicted to increase by 2.28 percentage points.
- If the incumbent party has only served one consecutive term, then the Incumbent Party Vote Share is predicted to increase by 4 percentage points.
2020 Prediction
The Time For Change Model is not a good predictor for the 2020 election.
Using our known variables for 2020:
- Q2 GDP Growth (%) = -9.4947159
- Net Approval Rating (%) = -15
- First Term = 1
We predict Trump’s Two-Party Vote Share:
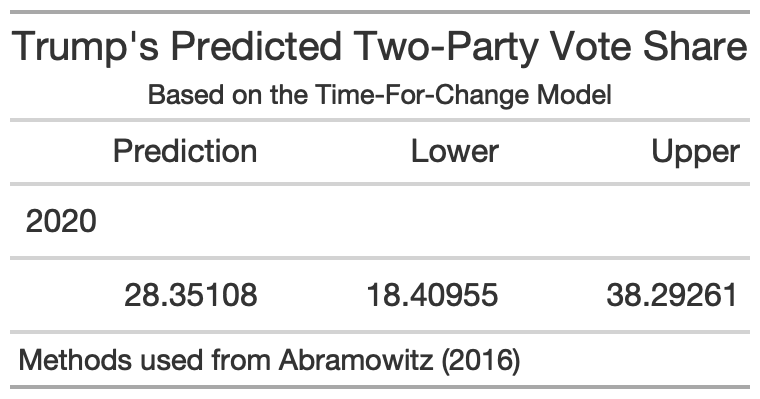
However, as noted before, the economy in 2020 is a huge outlier, because of the COVID-19 Pandemic. Because this model uses the economy as a predictor, we are extrapolating far outside the training set values, and thus we should be cautious. Because the Q2 GDP Growth is so low, this brings our prediction down significantly.
Abramowitz himself notes this in his article for UVA Center for Politics, and refines his own model with different variables. Using Net Approval in late October as his only predictor, Abramowitz now estimates the Electoral Vote instead of the Popular Vote for elections in which an Incumbent President is running.
Simplified Model
Using this same method we form a new simplified model, using the same Net Approval Rating data, and Presidential Election data from Wikipedia:
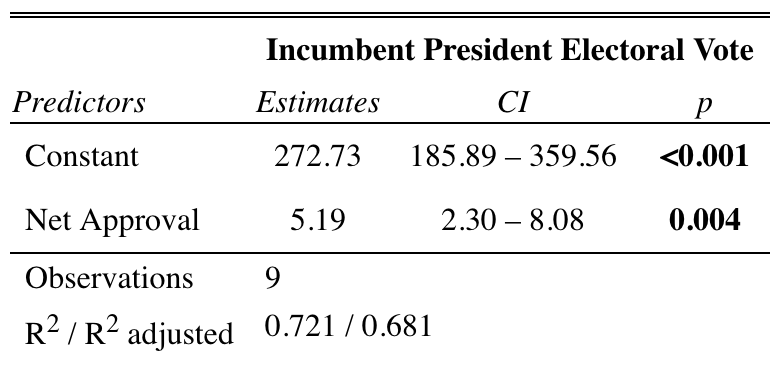
Using the coefficients, this gives us the equation:
(Predicted Incumbent Electoral Vote) = 272.73 + 5.19 * (Net Approval Rating)
This means that the model predicts for each additional percent in Net Approval Rating, an Incumbent President will earn about 5.19 more Electoral Votes.
It’s interesting to note that if the President’s Net Approval Rating were 0 (as many people disapproved of him as those who approved of him), the model predicts the slightest Electoral College victory for him (~273).
Simplified Model 2020 Prediction
For 2020, Trump’s most recent Net Approval Rating is -15%, so we get the following prediction:
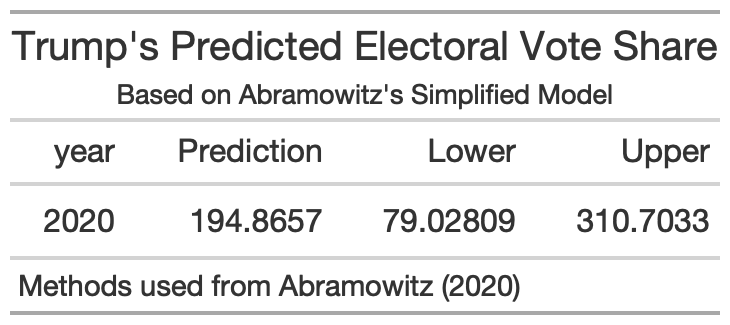
This predicts Trump will lose with ~195 Electoral Votes. However, the upper bound on the 95% Confidence Interval is ~310, which would be a win for Trump, so this model does not definitively predict a win for Biden.
Comparison to Polling Model
It seems that the Polling Model is a better predictor for the 2020 election, based on the fact that the Time For Change Model uses the economy as a predictor, which as discussed will not be too helpful for the 2020 election prediction.
However, the Simplified Model (using only Net Approval Ratings) does seem to do a moderately good job at predicting the electoral vote with an R-Squared Score of 0.66, which is not amazing, but not awful.
Since the Simplified Model predicts the electoral vote and the Polling Model predicts the popular vote, it is difficult to directly compare the two. However, they do both predict a Biden win.
In future models, it may be a good idea to possibly combine both of these models, by making them both predict the electoral vote or both predict the popular vote (or possibly both).